Introduction

Size is an important aspect of any visual stimulus, whether it is being viewed by a human out in the world or a by monkey in the laboratory. When studying vision, there is a crucial distinction between the size of an object in the world and the size the same object projects onto the retina. Object size in the world can be measured in any number of units: millimeters, inches, meters, miles, etc. Retinal size is measured in degrees of visual angle, a concept that incorporates both the size of the object and its distance from the observer. Calculating visual angle is less straightforward than you might think.

This activity will help you understand visual angle and how it is calculated.

Instructions

Click on the links above left to jump to different parts of the activity. Each part contains multiple images.

Click on the images to toggle between them (image numbers referred to in the explanations will appear in the upper area of the screen). The first time you do this activity, you should probably go through the parts in order from top to bottom.

What Is Visual Angle?

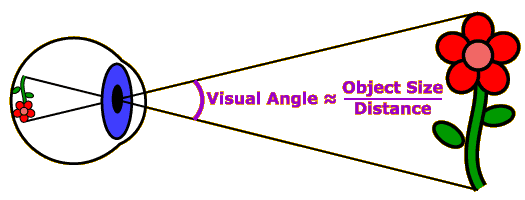
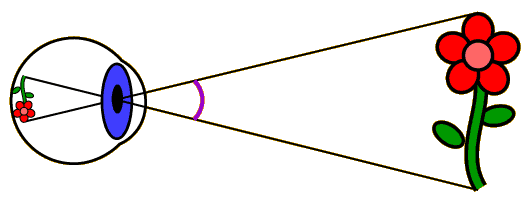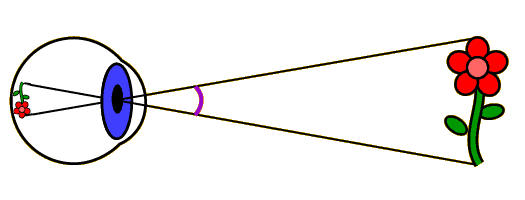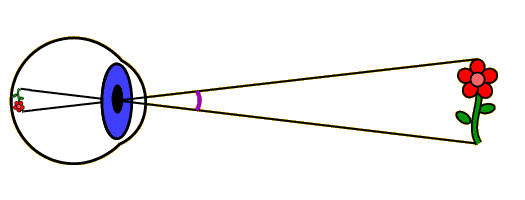
Image 1 shows an easy way to define visual angle. First, we draw lines connecting the top and bottom of a viewed object with the top and bottom of the projected image on the retina. As shown, the image passes through the pupil and is inverted (both top to bottom and left to right) on the back of the eye. The angle the two lines form as they cross each other is the visual angle. Visual angle is measured in degrees, with 360 degrees representing the entire circumference of the eyeball. The total visible area on the back of the eye for humans, the portion of the world that you can see, is about 180 degrees. A good guideline is that your thumb held at arm’s length is about two degrees across (we say that the image of the thumb subtends 2 degrees of visual angle).

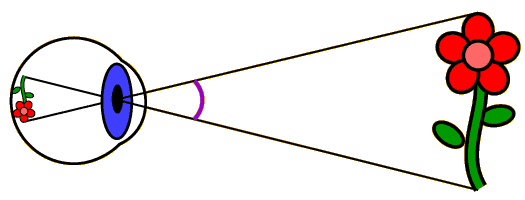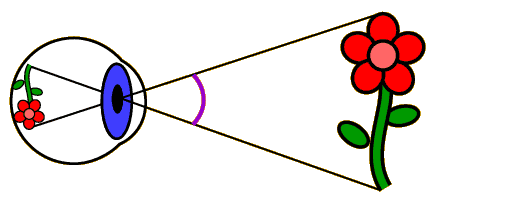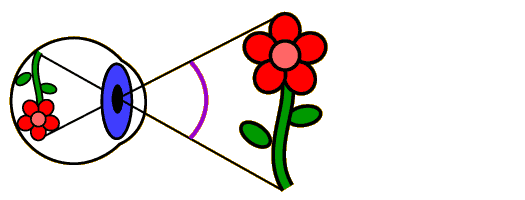
The formula in Image 1 states that visual angle is proportional to an object’s size divided by its distance from the eye (a more precise formula is given in the Visual Angle Calculator portion of the activity). It is important to understand this relationship: as an object gets closer to you, its visual angle becomes larger, as shown in Image 2. (You can also demonstrate this by starting with your thumb at arm’s length in front of you and then moving it halfway closer to your eye; now it subtends 4 degrees of visual angle instead of two.)

Additionally, for a given distance, as an object’s size decreases, its visual angle gets smaller, as shown in Image 3.

The Moon and Sun

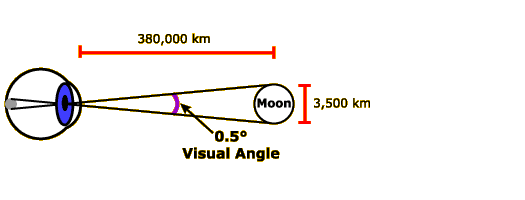
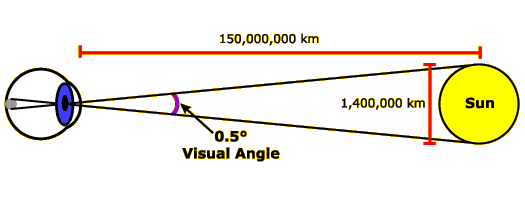
The moon provides a convenient illustration of visual angle because it is always about the same distance from every human observer (except astronauts and cosmonauts). As shown in Image 1, the moon is approximately 380,000 km from Earth, and its diameter is about 3,500 km. This works out to a visual angle of about 0.5 degrees (one-half a degree). By a cosmic coincidence, the sun also subtends about 0.5 degrees of visual angle, as shown in Image 2. The sun is much farther away than the moon, but its larger size perfectly compensates for the increase in distance and keeps the visual angle of the two heavenly bodies the same. This is why during a solar eclipse one can just see the outline of the sun behind the moon. If the moon had a much larger visual angle than the sun, one wouldn’t see the outline at all, whereas if the sun’s visual angle was larger, the moon would not completely cover it.

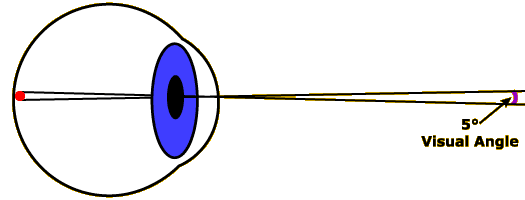
Note that these drawings are out of scale in several respects. Most importantly for the present discussion, one-half a degree of visual angle does not take up nearly as much of the retina as depicted in Images 1 and 2. Image 3 shows a more realistic drawing. Here, the red circle represents the approximate retinal area (relative to the size of the eyeball) taken up by an image subtending 5 degrees of visual angle (about 10 times the visual angle of the moon and sun). Therefore, the size of the moon and sun on your retina are actually quite tiny!

Visual Angle Calculator

Not satisfied with our statement that your outstretched thumb subtends “about” 2 degrees of visual angle? With the above tool you can calculate visual angles precisely. Measure the width of your thumb and the distance from your eye to your thumb at arm’s length and enter these values next to the words “Size” and “Distance” in the calculator. (It doesn’t matter whether you use inches or centimeters, as long as you use the same units for both measurements.) Then click the “Calculate” button next to the Visual Angle box, and the visual angle will appear.

Try entering the measurements for the moon and sun from the last part of the activity to confirm that these objects do in fact take up about 0.5 degrees of visual angle. You can also use this form to calculate size given visual angle and distance, or distance given visual angle and size. Just enter the two numbers you know in the appropriate boxes and click the “Calculate” button next to the box you don’t know.

For example, how large would an object have to be to subtend 5 degrees when it is 50 cm away from your eye? (Answer: 4.4 cm.)

A Visual Angle Illusion

Read all the instructions before starting this demonstration.

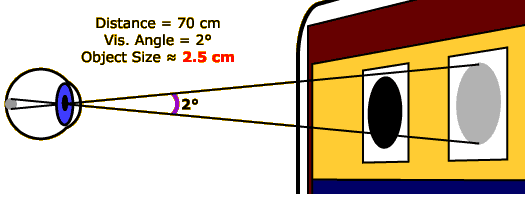
Have a blank piece of white paper in your hand. Then sit at a comfortable reading distance from the computer monitor (i.e., with your eyes about 70 cm from the screen) and stare at the center of the black circle above (Image 1) for about 30 seconds, without moving your eyes at all. This will cause you to see a negative color afterimage. Color afterimages will be discussed in Chapter 5, but for now, all you need to know is that staring at the black circle for a little while will cause you to see a white afterimage.

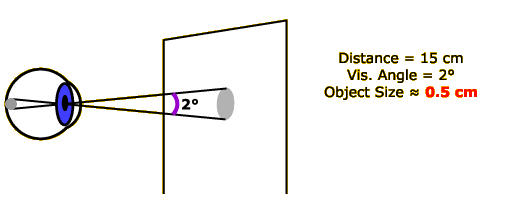
As a result, if you then shift your gaze to the white square to the right of the black circle, a ghostly shadow of the circle will appear (if the afterimage begins to fade, blink your eyes a few times and it may come back). Observe how big the afterimage appears to be. Then quickly hold the piece of paper about 15 cm in front of your eyes. The afterimage should reappear on the piece of paper. Interestingly, though, the afterimage should now appear to be much smaller than it did when you saw it on the computer monitor! You can also try looking at a distant wall across the room and observe that the circle afterimage appears to be quite large.

Why does the afterimage look smaller on the piece of paper than on the monitor or the wall?

Illusion Explained

Image 2 diagrams the initial conditions under which you viewed the afterimage. Assuming an average sized monitor and a viewing distance of 70 cm, the afterimage probably subtended 2 degrees of visual angle on your eye. When asked to guess the size of the circle, your brain did a quick calculation of the visual angle of the spot and the distance to the monitor and came up with an estimate of about 2.5 cm (yes, your visual system can do trigonometry without a calculator!). When you then held the paper in front of your eye, as shown in Image 3, your visual system detected that the apparent distance of the afterimage had changed. The visual angle of the circle, however, remained constant: remember that the afterimage works by bleaching out a circumscribed area of your retina, which will not change no matter what surface the afterimage is viewed against. Given these parameters, your visual system was forced to conclude that the circle was now only one-half a centimeter across. If you looked across the room at a distant wall, the circular afterimage appeared to be bigger because the wall is farther away from you than the monitor or paper. Therefore, your visual system concluded that the circle must have been quite large to take up the same amount of visual angle as it did when you were looking at the monitor or paper.

This illusion provides one of our first demonstrations that perception is sometimes far less precise than we usually believe it to be. When you view an object, all your brain really knows for sure is the visual angle the object occupies on the retina. Given this information alone, it is impossible to determine both the object’s size and distance. To solve this ill-defined problem, the visual system relies on rules, assumptions, and guesses about the world that usually serve us well, but sometimes break down and result in optical illusions.